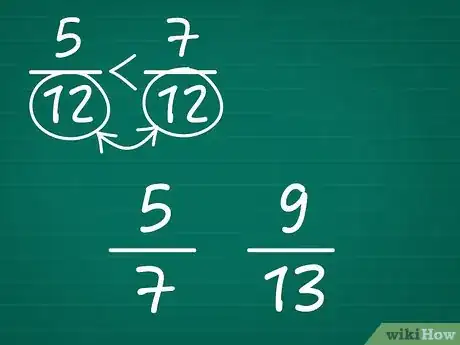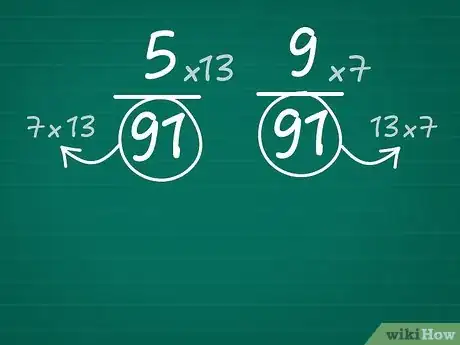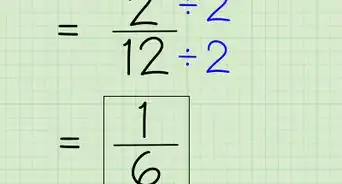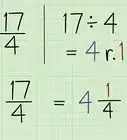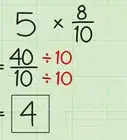This article was co-authored by Jake Adams. Jake Adams is an academic tutor and the owner of Simplifi EDU, a Santa Monica, California based online tutoring business offering learning resources and online tutors for academic subjects K-College, SAT & ACT prep, and college admissions applications. With over 14 years of professional tutoring experience, Jake is dedicated to providing his clients the very best online tutoring experience and access to a network of excellent undergraduate and graduate-level tutors from top colleges all over the nation. Jake holds a BS in International Business and Marketing from Pepperdine University.
This article has been viewed 51,272 times.
Comparing fractions means looking at two fractions and figuring out which one is greater. To compare fractions, all you have to do is to make it so that they have the same denominator and then see which fraction has the greater numerator -- this will tell you which fraction is greater. The tricky part is knowing how to make sure the fractions have like denominators, but it doesn't have to be so hard. If you want to know how to compare fractions, just follow these steps.
Steps
-
1Determine whether or not the fractions have the same denominator. This is the first step to comparing fractions. The denominator is the number on the bottom of the fraction and the numerator is the number on top.[1] For example, the fractions 5/7 and 9/13 do not have the same denominator, because 7 does not equal 13, so you'll have to take a few steps to compare them.[2]
- If the denominator of the fractions is the same, then all you have to do is look at the numerator to know which fraction is greater. For example, with the fraction 5/12 and 7/12, you know that 7/12 is greater than 5/12 because 7 is greater than 5.
-
2Find a common denominator. To be able to compare the fractions, you'll need to find a common denominator so you can figure out which fraction is greater.[3] If you were adding and subtracting fractions with unlike denominators, then it would be best to find the least common denominator for the fractions. But since you're just comparing the fractions, you can just take a shortcut and multiply the denominators of both fractions to find the common denominator.
- 7 x 13 = 91, so the new denominator will be 91.
Advertisement -
3Change the numerators of the fractions.[4] Now that you've changed the denominators of the fractions to 91, you'll need to change the numerators so the value of the fractions remains the same. To do this, you'll need to multiply the numerator of each fraction by the same number that you multiplied the denominator by to get 91. Here's how you do it:
- With the original fraction 5/7, you multiplied 7 by 13 to get a new denominator of 91, so you'll need to multiply 5 by 13 to get the new numerator. You're essentially multiplying both the numerator and the denominator of the fraction by 13/13 (which equals 1). 5/7 x 13/13 = 65/91.
- With the original fraction 9/13, you multiplied 13 by 7 to get a new denominator of 91, so you'll need to multiply 9 by 7 to get the new numerator. 9 x 7 = 63, so the new fraction is 63/91.
-
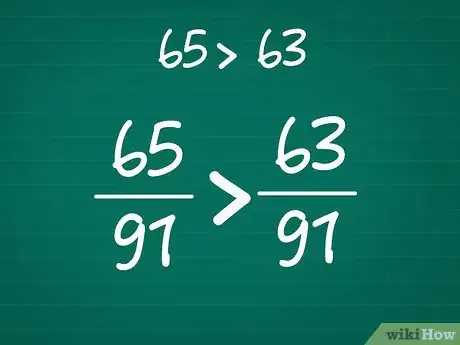
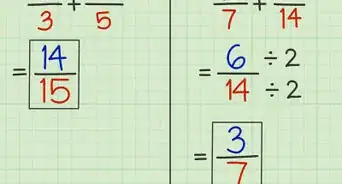
4Compare the numerators of the fractions. The one with the larger numerator is the greater fraction.[5] So, the fraction 65/91 is greater than 63/91 because 65 is greater than 63. This means that the original fraction, 5/7, is greater than 9/13.
Expert Q&A
-
QuestionHow do you introduce fractions in lessons?
Jake AdamsJake Adams is an academic tutor and the owner of Simplifi EDU, a Santa Monica, California based online tutoring business offering learning resources and online tutors for academic subjects K-College, SAT & ACT prep, and college admissions applications. With over 14 years of professional tutoring experience, Jake is dedicated to providing his clients the very best online tutoring experience and access to a network of excellent undergraduate and graduate-level tutors from top colleges all over the nation. Jake holds a BS in International Business and Marketing from Pepperdine University.
Academic Tutor & Test Prep SpecialistUse pies to represent fractions! Try to get students to understand that the greater the number of slices in that pie (which represents the fraction denominator), the smaller the slice of the pie. For instance, 1/2 is going to be bigger than 1/100, because the 2 on the bottom is smaller. It also helps to show them how to get matching denominators through multiplication on the top and bottom of the fraction. -
QuestionWhy do I need to compare the denominator of dissimilar fractions?
DonaganTop AnswererYou don't compare denominators. You convert the dissimilar fractions to equivalent fractions that have identical denominators. Then you compare numerators. -
QuestionI'm a student and I want to know the steps to divide decimals. What are the steps on how to use models to write fractions as a decimal?
Community AnswerSee the wikiHow article on how to convert fractions to decimals.
Expert Interview
Thanks for reading our article! If you'd like to learn more about learning mathematics, check out our in-depth interview with Jake Adams.
References
- ↑ https://www.mathsisfun.com/definitions/denominator.html
- ↑ http://www.mathsisfun.com/comparing-fractions.html
- ↑ https://www.mathsisfun.com/numbers/common-denominator.html
- ↑ https://www.khanacademy.org/math/arithmetic/fraction-arithmetic/arith-review-comparing-fractions/v/comparing-fractions-2
- ↑ https://www.montereyinstitute.org/courses/DevelopmentalMath/TEXTGROUP-1-8_RESOURCE/U02_L1_T5_text_final.html
About This Article
To compare fractions, or tell if one fraction is bigger than another, check if the fractions have the same denominator. If they do, just see which numerator is bigger. If not, you can make both denominators the same by multiplying them together–this is called finding a common denominator. Once you’ve made both denominators equal, multiply each numerator by the same number that you multiplied its denominator by. Then just compare the numerators to see which fraction is bigger!
