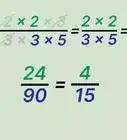This article was co-authored by David Jia. David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.
There are 7 references cited in this article, which can be found at the bottom of the page.
This article has been viewed 369,651 times.
Two fractions are equivalent if they have the same value. Knowing how to convert a fraction into an equivalent one is an essential math skill that's necessary for everything from basic algebra to advanced calculus. This article will cover several ways to calculate equivalent fractions from basic multiplication and division to more complex methods for solving equivalent fraction equations.
Steps
Forming Equivalent Fractions
-
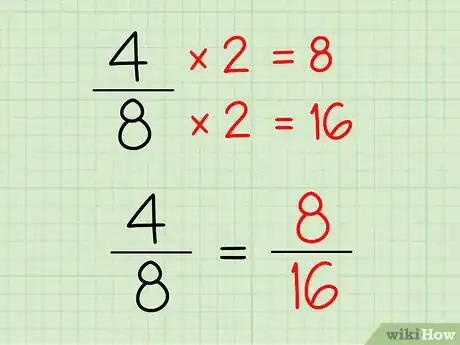
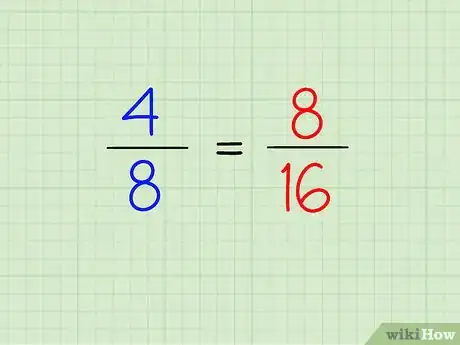
1Multiply the numerator and denominator by the same number. Two fractions that are different but equivalent have, by definition, numerators and denominators that are multiples of each other. In other words, multiplying the numerator and denominator of a fraction by the same number will produce an equivalent fraction. Though the numbers in the new fraction will be different, the fractions will have the same value.[1]
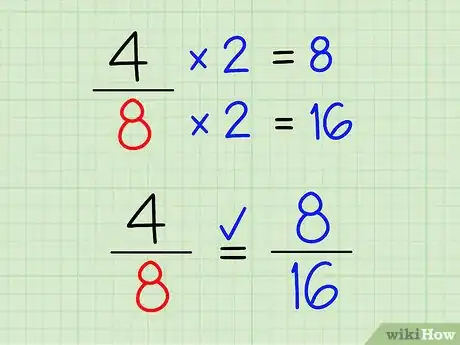
- For instance, if we take the fraction 4/8 and multiply both the numerator and denominator by 2, we get (4×2)/(8×2) = 8/16. These two fractions are equivalent.
- (4×2)/(8×2) is essentially the same as 4/8 × 2/2 Remember that when multiplying two fractions, we multiply across, meaning numerator to numerator and denominator to denominator.
- Notice that 2/2 equals 1 when you carry out the division. Thus, it's easy to see why 4/8 and 8/16 are equivalent since multiplying 4/8 × (2/2) = 4/8 still. The same way it’s fair to say that 4/8 = 8/16.
- Any given fraction has an infinite number of equivalent fractions. You can multiply the numerator and denominator by any whole number, no matter how large or small to obtain an equivalent fraction.
-
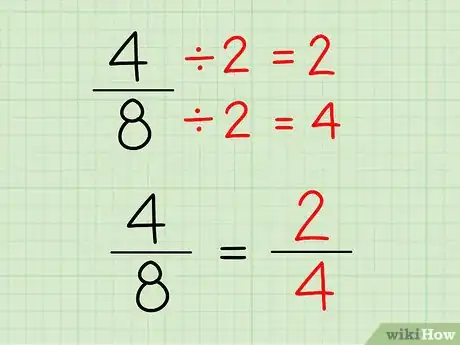
2Divide the numerator and denominator by the same number. Like multiplication, division can also be used to find a new fraction that's equivalent to your starting fraction. Simply divide the numerator and the denominator of a fraction by the same number to obtain an equivalent fraction. There is one caveat to this process--the resulting fraction must have whole numbers in both the numerator and denominator to be valid.[2]
- For instance, let's look at 4/8 again. If, instead of multiplying, we divide both the numerator and denominator by 2, we get (4 ÷ 2)/(8 ÷ 2) = 2/4. 2 and 4 are both whole numbers, so this equivalent fraction is valid.
Using Basic Multiplication to Determine Equivalency
-
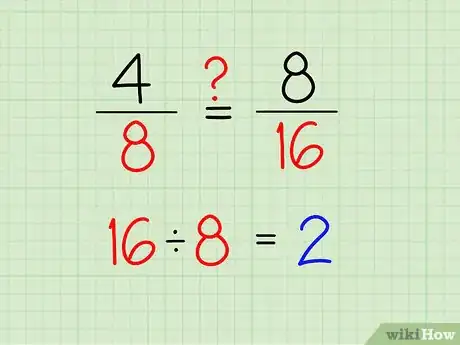
1Find the number by which the smaller denominator needs to be multiplied to make the larger denominator. Many problems regarding fractions involve determining if two fractions are equivalent. By calculating this number, you can begin putting the fractions in the same terms to determine equivalency.[3]
- For example, take the fractions 4/8 and 8/16 again. The smaller denominator is 8, and we would have to multiply that number x2 in order to make the larger denominator, which is 16. Therefore, the number in this case is 2.[4]
- For more difficult numbers, you can simply divide the larger denominator by the smaller denominator. In this case 16 divided by 8, which still gets us 2.
- The number may not always be a whole number. For example, if the denominators were 2 and 7, then the number would be 3.5.
-
2Multiply the numerator and denominator of the fraction expressed in lower terms by the number from the first step. Two fractions that are different but equivalent have, by definition, numerators and denominators that are multiples of each other. In other words, multiplying the numerator and denominator of a fraction by the same number will produce an equivalent fraction. Though the numbers in this new fraction will be different, the fractions will have the same value.[5]
- For instance, if we take the fraction 4/8 from step one and multiply both the numerator and denominator by our previously determined number 2, we get (4×2)/(8×2) = 8/16. Thus proving that these two fractions are equivalent.
Using Basic Division to Determine Equivalency
-
1Calculate each fraction as a decimal number. For simple fractions without variables, you can simply express each fraction as a decimal number to determine equivalency. Since every fraction is actually a division problem to begin with, this is the simplest way to determine equivalency.[6]
- For instance, take our previously used 4/8. The fraction 4/8 is equivalent to saying 4 divided by 8, which 4/8 = 0.5. You can solve for the other example as well, which is 8/16 = 0.5. Regardless of the terms of a fraction, they are equivalent if the two numbers are exactly the same when expressed as a decimal.
- Remember that the decimal expression may go several digits before the lack of equivalence becomes apparent. As a basic example, 1/3 = 0.333 repeating while 3/10 = 0.3. By using more than one digit, we see that these two fractions are not equivalent.
-
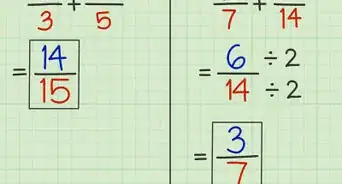
2Divide the numerator and denominator of a fraction by the same number to get an equivalent fraction. For more complex fractions, the division method requires additional steps. As with the multiplication method, you can divide the numerator and the denominator of a fraction by the same number to obtain an equivalent fraction. There is one caveat to this process. The resulting fraction must have whole numbers in both the numerator and denominator to be valid.[7]
- For instance, let's look at 4/8 again. If, instead of multiplying, we divide both the numerator and denominator by 2, we get (4 ÷ 2)/(8 ÷ 2) = 2/4. 2 and 4 are both whole numbers, so this equivalent fraction is valid.
-
3Reduce the fractions to their lowest terms. Most fractions should typically be expressed in their lowest terms, and you can convert fractions to their simplest terms by dividing by their greatest common factor (GCF).[8] This step operates by the same logic of expressing equivalent fractions by converting them to have the same denominator, but this method seeks to reduce each fraction to its lowest expressible terms.
- When a fraction is in its simplest terms, its numerator and denominator are both as small as they can be. Neither can be divided by the any whole number to obtain anything smaller. To convert a fraction that's not in simplest terms to an equivalent form that is, we divide the numerator and denominator by their greatest common factor.
- The greatest common factor (GCF) of the numerator and denominator is the largest number that divides into both to give a whole number result. So, in our 4/8 example, since 4 is the largest number that divides evenly into both 4 and 8, we would divide the numerator and denominator of our fraction by 4 to get it in simplest terms. (4 ÷ 4)/(8 ÷ 4) = 1/2. For our other example of 8/16, the GCF is 8, which also results in 1/2 as the simplest expression of the fraction.
Using Cross Multiplication to Solve for a Variable
-
1Set the two fractions equal to one another. We use cross multiplication for math problems where we know the fractions are equivalent, but one of the numbers has been replaced with a variable (typically x) for which we must solve. In cases like this, we know these fractions are equivalent because they're the sole terms on opposite sides of an equal sign, but it's often not obvious how to solve for the variable. Luckily, with cross multiplication, solving these types of problems is easy.[9]
-
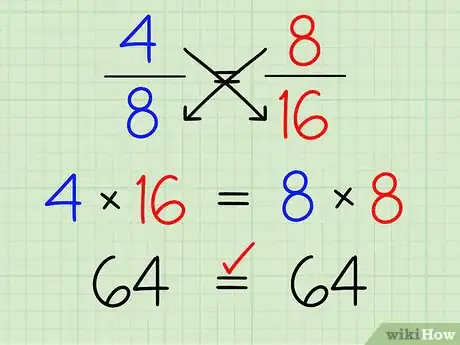
2Take the two equivalent fractions and multiply across the equals sign in an "X" shape. In other words, you multiply the numerator of one fraction by the denominator of the other and vice versa, then set these two answers equal to each other and solve.[10]
- Take our two examples of 4/8 and 8/16. These two don't contain a variable, but we can prove the concept since we already know they're equivalent. By cross multiplying, we get 4 x 16 = 8 x 8, or 64 = 64, which is obviously true. If the two numbers are not the same, then the fractions are not equivalent.
-
3Introduce a variable. Since cross multiplication is the easiest way to determine equivalent fractions when you must solve for a variable, let's add a variable.
- For example, let's consider the equation 2/x = 10/13. To cross multiply, we multiply 2 by 13 and 10 by x, then set our answers equal to each other:
- 2 × 13 = 26
- 10 × x = 10x
- 10x = 26. From here, getting an answer for our variable is a matter of simple algebra. x = 26/10 = 2.6, making the initial equivalent fractions 2/2.6 = 10/13.
- For example, let's consider the equation 2/x = 10/13. To cross multiply, we multiply 2 by 13 and 10 by x, then set our answers equal to each other:
-
4Use cross multiplication for equations with multiple variables or variable expressions. One of the best things about cross multiplication is that it works in essentially the same way whether you're dealing with two simple fractions (as above) or with more complex fractions. For instance, if both fractions contain variables, you just have to eliminate these variables at the end during the solving process. Similarly, if the numerators or denominators of your fractions contain variable expressions (such as x + 1), simply "multiply through"by using the distributive property and solve as you normally would.[11]
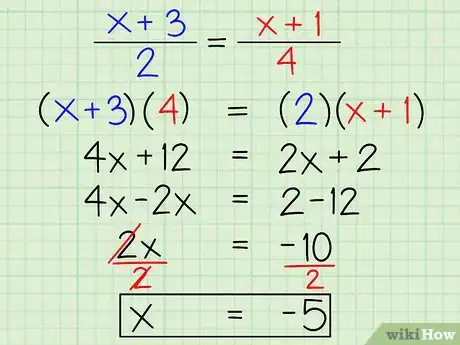
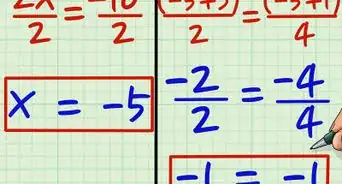
- For instance, let's consider the equation ((x + 3)/2) = ((x + 1)/4). In this case, as above, we'll solve by cross multiplying:
- (x + 3) × 4 = 4x + 12
- (x + 1) × 2 = 2x + 2
- 2x + 2 = 4x + 12, then we can simplify the equation by subtracting 2x from both sides
- 2 = 2x + 12, then we should isolate the variable by subtracting 12 from both sides
- -10 = 2x, and divide by 2 to solve for x
- -5 = x
- For instance, let's consider the equation ((x + 3)/2) = ((x + 1)/4). In this case, as above, we'll solve by cross multiplying:
Using the Quadratic Formula to Solve for Variables
-
1Cross multiply the two fractions. For equivalency problems that require the quadratic formula, we still begin by using cross multiplication. However, any cross multiplication that involves multiplying variable terms by other variable terms is likely to result in an expression that can't easily be solved via algebra. In cases like these, you may need to use techniques like factoring and/or the Quadratic Formula.[12]
- For example, let's look at the equation ((x +1)/3) = (4/(2x - 2)). First, let's cross multiply:
- (x + 1) × (2x - 2) = 2x2 + 2x -2x - 2 = 2x2 - 2
- 4 × 3 = 12
- 2x2 - 2 = 12.
- For example, let's look at the equation ((x +1)/3) = (4/(2x - 2)). First, let's cross multiply:
-
2Express the equation as a quadratic equation. At this point, we want to express this equation in quadratic form (ax2 + bx + c = 0), which we do by setting the equation equal to zero.[13] In this case, we subtract 12 from both sides to get 2x2 - 14 = 0.
- Some values may equal 0. Though 2x2 - 14 = 0 is the simplest form of our equation, the true quadratic equation is 2x2 + 0x + (-14) = 0. It will probably help early on to mirror the form of the quadratic equation even when some values are 0.
-
3Solve by plugging the numbers from your quadratic equation into the quadratic formula. The quadratic formula (x = (-b +/- √(b2 - 4ac))/2a) will help us solve for our value x at this point.[14] Don’t be intimidated by the length of the formula. You’re simply taking the values from your quadratic equation in step two and plugging them into the appropriate spots before solving.
- x = (-b +/- √(b2 - 4ac))/2a. In our equation, 2x2 - 14 = 0, a = 2, b = 0, and c = -14.
- x = (-0 +/- √(02 - 4(2)(-14)))/2(2)
- x = (+/- √( 0 - -112))/2(2)
- x = (+/- √(112))/2(2)
- x = (+/- 10.58/4)
- x = +/- 2.64
-
4Check your answer by plugging the x value back into your quadratic equation. By plugging the calculated value of x back into your quadratic equation from step two, you can easily determine if you reached the correct answer.[15] In this example, you would plug both 2.64 and -2.64 into the original quadratic equation.
Expert Q&A
-
QuestionHow can I use multiplication to find equivalent fractions?
 David JiaDavid Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.
David JiaDavid Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.
Academic Tutor Multiply the top number and bottom number by the same value to find a fraction that's equivalent to the original.
Multiply the top number and bottom number by the same value to find a fraction that's equivalent to the original. -
QuestionHow do I draw a picture to show that 1/3 is equivalent to 2/6?
 DonaganTop AnswererAn easy way is to draw a circle graph. Divide the circle into sixths. Each sixth of the circle would have a central angle of 60°. Then show (perhaps with the use of color) that two adjacent sixths combine to make one-third of the circle (with a central angle of 120°).
DonaganTop AnswererAn easy way is to draw a circle graph. Divide the circle into sixths. Each sixth of the circle would have a central angle of 60°. Then show (perhaps with the use of color) that two adjacent sixths combine to make one-third of the circle (with a central angle of 120°). -
QuestionWhat is equivalent to 4 and 2/14?
 DonaganTop AnswererFor easier computation, convert 2/14 to 1/7. So the mixed number is 4 1/7. If you want to convert that number to an improper fraction, keep the denominator the same. For the new numerator, multiply the whole number by the denominator and add the old numerator. In this example, multiply 4 by 7 to get 28, then add 1 to get a numerator of 29. The denominator is 7.
DonaganTop AnswererFor easier computation, convert 2/14 to 1/7. So the mixed number is 4 1/7. If you want to convert that number to an improper fraction, keep the denominator the same. For the new numerator, multiply the whole number by the denominator and add the old numerator. In this example, multiply 4 by 7 to get 28, then add 1 to get a numerator of 29. The denominator is 7.
Warnings
- Multiplication and division work for obtaining equivalent fractions because multiplying and dividing by fractional forms of the number 1 (2/2, 3/3, etc.) give answers that are equivalent to the starting fraction by definition. Addition and subtraction don't allow this possibility.⧼thumbs_response⧽
- Although you multiply the numerators and denominators together when multiplying fractions, you do not add or subtract denominators when adding or subtracting fractions.
- For example, above, we found that 4/8 ÷ 4/4 = 1/2 . If we instead added by 4/4, we would have gotten a completely different answer. 4/8 + 4/4 = 4/8 + 8/8 = 12/8 = 1 1/2 or 3/2, neither of which are equal to 4/8.
⧼thumbs_response⧽
References
- ↑ https://www.mathsisfun.com/equivalent_fractions.html
- ↑ https://www.cuemath.com/numbers/equivalent-fractions/
- ↑ https://www.mathsisfun.com/equivalent_fractions.html
- ↑ David Jia. Academic Tutor. Expert Interview. 23 February 2021
- ↑ http://www.themathpage.com/arith/equivalent-fractions.htm
- ↑ https://www.cuemath.com/numbers/equivalent-fractions/
- ↑ https://www.mathsisfun.com/equivalent_fractions.html
- ↑ David Jia. Academic Tutor. Expert Interview. 23 February 2021
- ↑ http://www.helpwithfractions.com/math-homework-helper/equivalent-fractions/
- ↑ http://www.helpwithfractions.com/math-homework-helper/equivalent-fractions/
- ↑ https://www.cuemath.com/numbers/equivalent-fractions/
- ↑ http://www.purplemath.com/modules/solvquad4.htm
- ↑ https://www.khanacademy.org/math/algebra/x2f8bb11595b61c86:quadratic-functions-equations/x2f8bb11595b61c86:quadratic-formula-a1/a/quadratic-formula-review
- ↑ http://www.purplemath.com/modules/solvquad4.htm
- ↑ http://www.purplemath.com/modules/solvquad4.htm
About This Article
To find equivalent fractions, multiply or divide the numerator and the denominator of a fraction by any number, as long as it’s the same on top and bottom. If you divide the original fraction, the result must have whole numbers in the numerator and denominator to be valid. To check the result, use cross-multiplication. Multiply the denominator of the first fraction by the numerator of the second fraction, then multiply the first numerator by the second denominator. If the 2 answers are equal, the fractions are equivalent. If you need to learn how to solve variables in your fraction, keep reading the article!

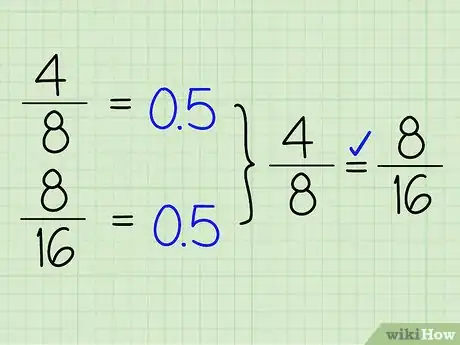
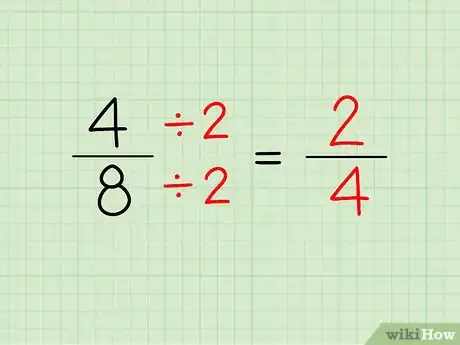
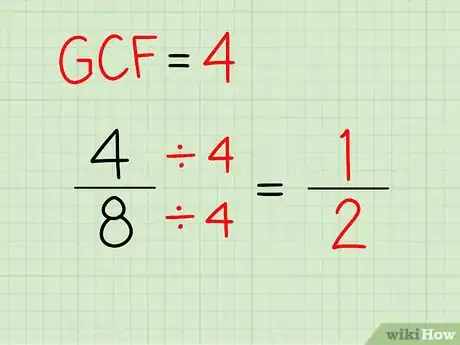
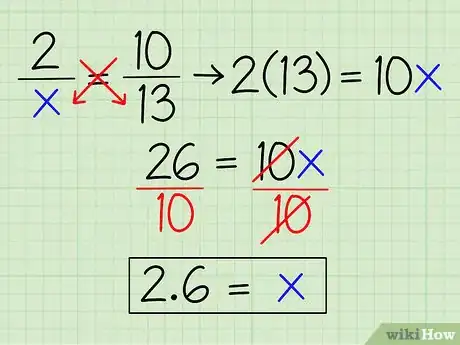
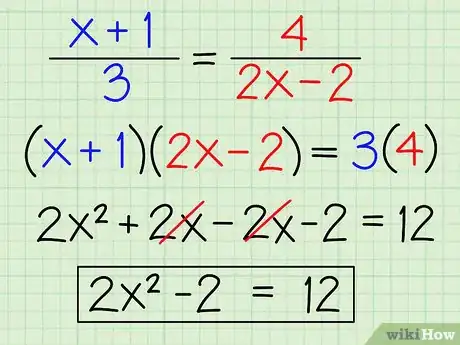
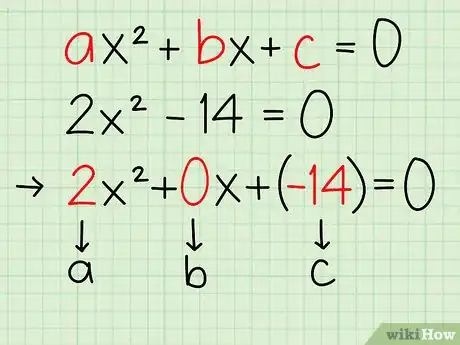
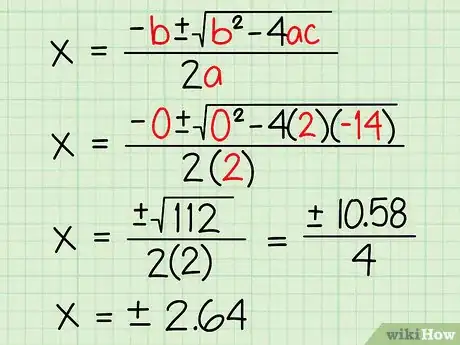

-Step-31-Version-2.webp)


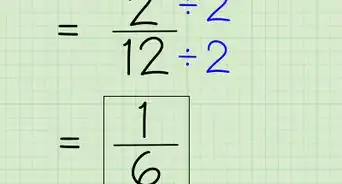









-Step-31-Version-2.webp)