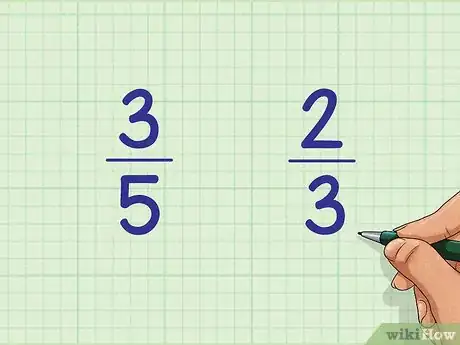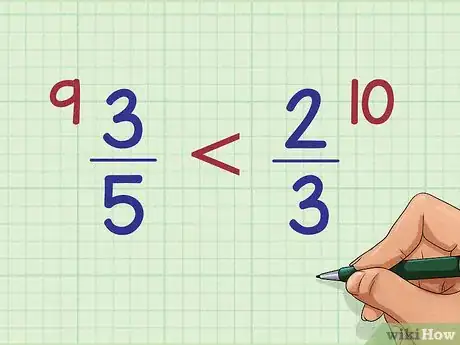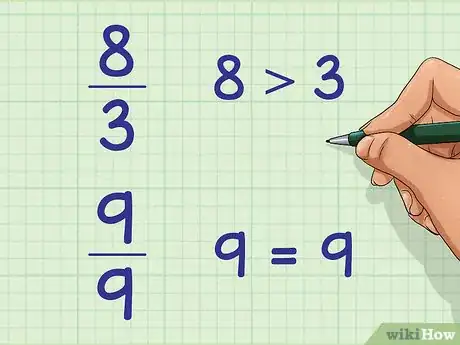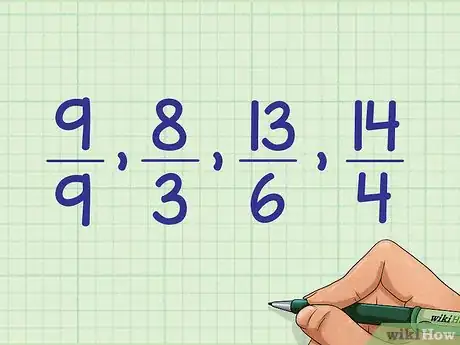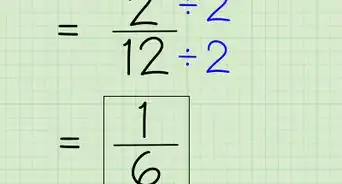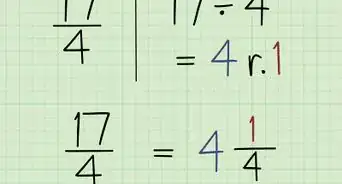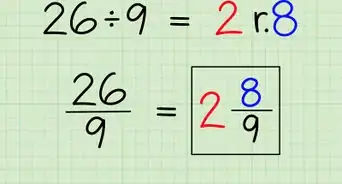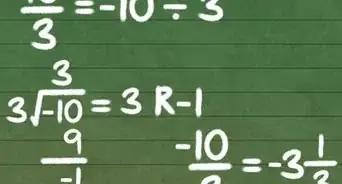How to Order Fractions From Least to Greatest

Co-authored by David Jia

Last Updated: August 24, 2021 References

While it's easy to order whole numbers like 1, 3, and 8 by size, fractions can be hard to measure at a glance. If each lower number, or denominator, is the same, you can order them like whole numbers, for instance 1/5, 3/5, and 8/5. Otherwise, you can alter your list of fractions to use the same denominator, without changing the size of any fraction. This becomes easier with practice, and you can learn a couple "tricks" as well when comparing just two fractions, or when you're sorting top-heavy "improper" fractions like 7/3.

Method 1

Method 1 of 3:

Ordering Any Number of Fractions

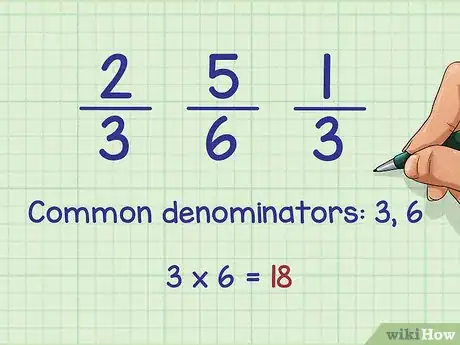
1
Find a common denominator for all the fractions. Use one of these methods to find a denominator, or lower number of a fraction, that you can use to rewrite every fraction in the list, so you can easily compare them. This is called a common denominator, or the lowest common denominator if it is the lowest one possible:^{[1]
X
Research source}
- Multiply every different denominator together. For example, if you are comparing 2/3, 5/6, and 1/3, multiply the two different denominators: 3 x 6 = 18. This is a simple method, but will often result in a much larger number than the other methods, which can be difficult to work with.^{[2]
  X
  Expert Source
  
  David Jia
  Academic Tutor
  Expert Interview. 23 February 2021}
- Or list the multiples of each denominator in a separate column, until you notice a number that shows up on every column. Use this number. For example, comparing 2/3, 5/6, and 1/3, list a few multiples of 3: 3, 6, 9, 12, 15, 18. Then list the multiples of 6: 6, 12, 18. Since 18 shows up on both lists, use that number. (You could also use 12, but the examples below will assume you are using 18.)
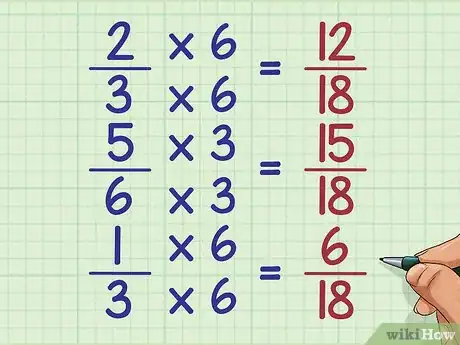
2
Convert each fraction so it uses the common denominator. Remember, if you multiply a fraction's top and bottom by the same amount, the fraction is still the same size.^{[3]
X
Expert Source

David Jia
Academic Tutor
Expert Interview. 23 February 2021} Use this technique on each fraction, one by one, so that each one uses the common denominator as the bottom number. Try it for 2/3, 5/6, and 1/3, using the common denominator 18:
- 18 ÷ 3 = 6, so 2/3 = (2x6)/(3x6)=12/18
- 18 ÷ 6 = 3, so 5/6 = (5x3)/(6x3)=15/18
- 18 ÷ 3 = 6, so 1/3 = (1x6)/(3x6)=6/18
Advertisement
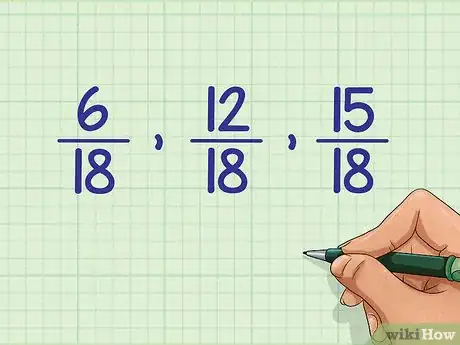
3
Use the top number to order the fractions. Now that they all have the same denominator, the fractions are easy to compare. Use their top number, or numerator, to rank them from least to greatest. Ranking the fractions we found above, we get: 6/18, 12/18, 15/18.
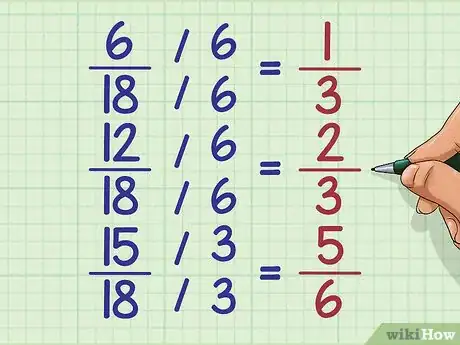
4
Return each fraction to its original form. Keep the fractions in the same order, but return each one back to its original form. You can do this by remembering how each fraction transformed, or by dividing the top and bottom of each fraction again:
- 6/18 = (6 ÷ 6)/(18 ÷ 6) = 1/3
- 12/18 = (12 ÷ 6)/(18 ÷ 6) = 2/3
- 15/18 = (15 ÷ 3)/(18 ÷ 3) = 5/6
- The answer is "1/3, 2/3, 5/6"

Method 2

Method 2 of 3:

Ordering Two Fractions using Cross-Multiplication

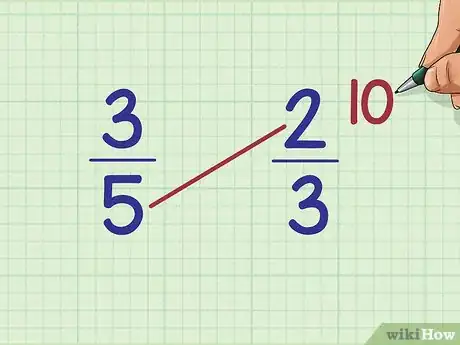
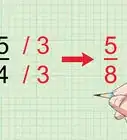
1
Write the two fractions next to each other. For example, compare the fraction 3/5 and the fraction 2/3. Write these next to each other on the page: 3/5 on the left, and 2/3 on the right.
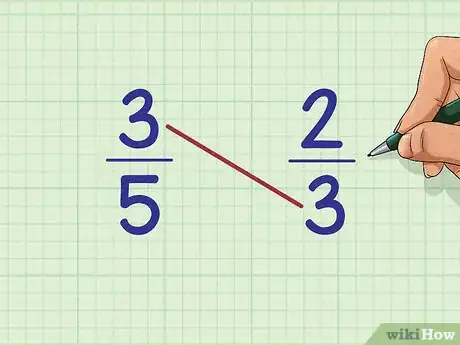
2
Multiply the top of the first fraction with the bottom of the second fraction. In our example, the top number, or numerator, of the first fraction (3/5) is 3. The bottom number, or denominator, of the second fraction (2/3) is also 3. Multiply these together: 3 x 3 = ?
- This method is called cross-multiplication, because you multiply numbers in a diagonal line across from each other.
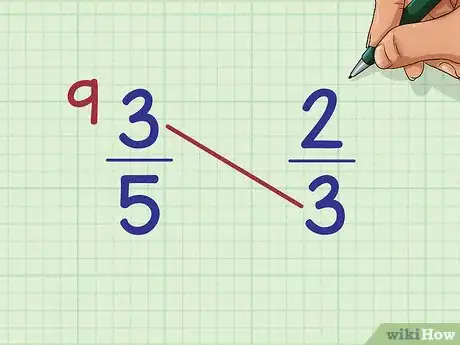
3
Write your answer next to the first fraction. Write the product, or answer to your multiplication problem, next to the first fraction on the page. In our example, 3 x 3 = 9, so you would write 9 next to the first fraction, on the left side of the page.
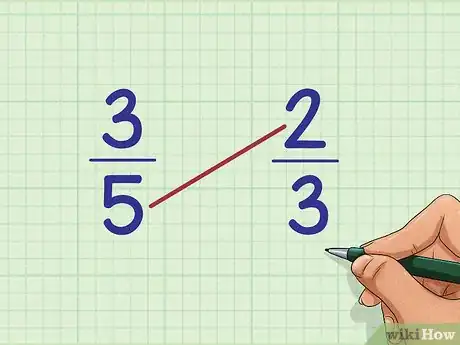
4
Multiply the top of the second fraction with the bottom of the first. To find out which fraction is larger, we'll need to compare our answer above with the answer to another multiplication problem. Multiply these two numbers together. For our example (comparing 3/5 and 2/3), multiply 2 x 5 together.
5
Write this answer next to the second fraction. Write the answer to this second multiplication problem next to the second fraction. In this example, the answer is 10.
6
Compare the values of the two cross-products. The answers to the multiplication problems in this method are called cross-products. If one cross-product is larger than the other, then the fraction next to that cross-product is also larger than the other fraction. In our example, because 9 is less than 10, this means 3/5 must be less than 2/3.
- Remember, always write the cross-product next to the fraction whose top number you used.
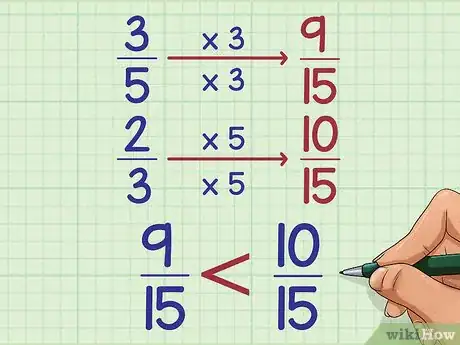
7
Understand why this works. To compare two fractions, typically you transform them to give them the same denominator, or lower part of the fraction. Secretly, this is what cross-multiplication does!^{[4]
X
Research source} It just skips over actually writing the denominators, since once the two fractions have the same one, you only need to compare the top two numbers. Here's our same example (3/5 vs 2/3), written without the cross-multiplying "shortcut":
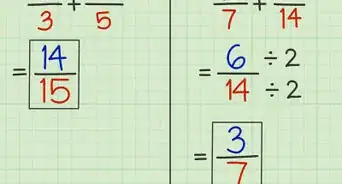
- 3/5=(3x3)/(5x3)=9/15
- 2/3=(2x5)/(3x5)=10/15
- 9/15 is less than 10/15
- Therefore, 3/5 is less than 2/3

Method 3

Method 3 of 3:

Ordering Fractions Larger than One

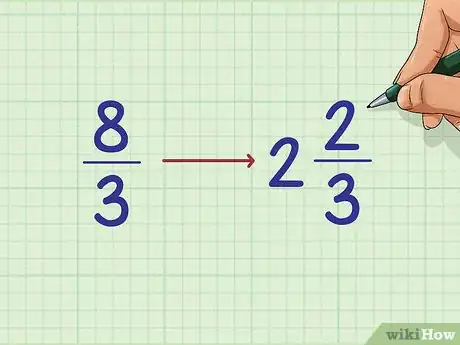
1
Use this for fractions with a top number equal or larger than the bottom number. If a fraction has a top number, or numerator, that is larger than the bottom number, or denominator, it is larger than one. 8/3 is one example of this type of fraction. You can also use this for fractions with an equal numerator and denominator, such as 9/9. Both of these fractions are examples of improper fractions.^{[5]
X
Research source}
- You can still use the other methods for these fractions. This method helps these fractions make sense, however, and might be faster.
2
Convert each improper fraction into a mixed number. Turn them into a mix of whole numbers and fractions. Sometimes, you might be able to do this in your head. For example, 9/9 = 1. Other times, use long division to find out how many times the numerator goes evenly into the denominator. The remainder in that long division problem, if any, gets "left over" as a fraction. For instance:
- 8/3 = 2 + 2/3
- 9/9 = 1
- 19/4 = 4 + 3/4
- 13/6 = 2 + 1/6
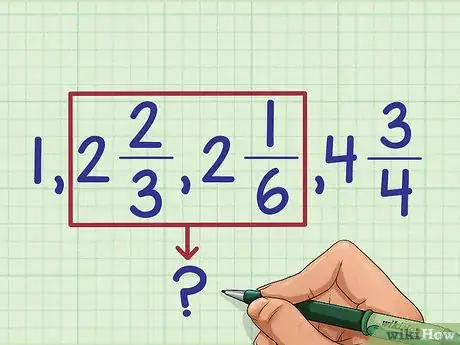
3
Sort the mixed numbers by whole number. Now that there are no improper fractions, you have a better idea of how large each number is. Ignore the fractions for now, and sort the fractions into groups by whole number:
- 1 is the smallest
- 2 + 2/3 and 2 + 1/6 (we don't yet know which is larger than the other)
- 4 + 3/4 is the largest
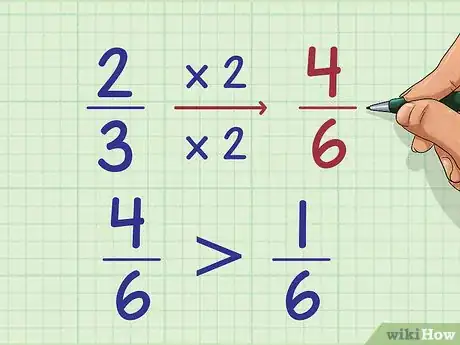
4
If necessary, compare the fractions in each group. If you have multiple mixed numbers with the same whole number, such as 2 + 2/3 and 2 + 1/6, compare the fraction part of the number to see which is larger. You can use any of the methods in the other sections to do this. Here's an example comparing 2 + 2/3 and 2 + 1/6, converting the fractions to the same denominator:
- 2/3 = (2x2)/(3x2) = 4/6
- 1/6 = 1/6
- 4/6 is greater than 1/6
- 2 + 4/6 is greater than 2 + 1/6
- 2 + 2/3 is greater than 2 + 1/6
5
Use your results to sort your whole list of mixed numbers. Once you've sorted the fractions in each group of mixed numbers, you can sort your entire list: 1, 2 + 1/6, 2 + 2/3, 4 + 3/4.
6
Convert the mixed numbers back to their original fractions. Keep the order the same, but undo the changes you made and write the numbers as the original improper fractions: 9/9, 8/3, 13/6, 19/4.

Expert Q&A

Question

How can I tell which fraction is greater?

David Jia
Academic Tutor
David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.

David Jia

Academic Tutor

Expert Answer

Look for a common denominator between the fractions, then multiply the numerators and denominators by the same value. Then you can see which fraction is greater just by the numerator.
Question

Which is the lowest: 3/5, 3/4, 4/7, or 2/3?

Jasmine Tipping

Community Answer

4/7 is the lowest, then 3/5, 2/3 and 3/4.
Question

Can I convert them into decimals while ordering them?

Community Answer

Yes you can, the order will be the same. Just make sure to convert back to fractions for your final answer if the original numbers are given as fractions.