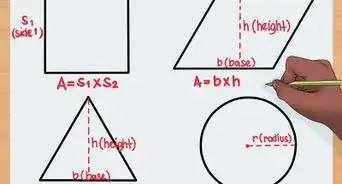
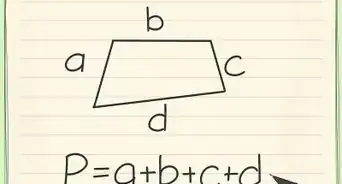
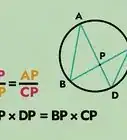
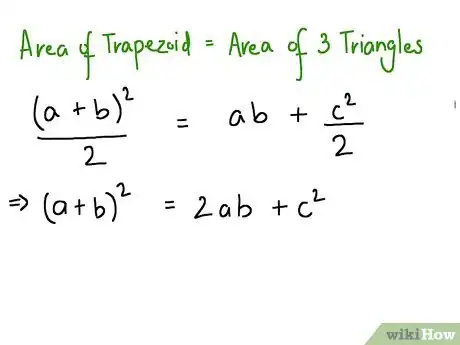X
wikiHow is a “wiki,” similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, 13 people, some anonymous, worked to edit and improve it over time.
This article has been viewed 141,947 times.
Learn more...
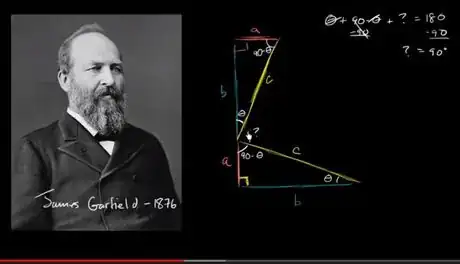
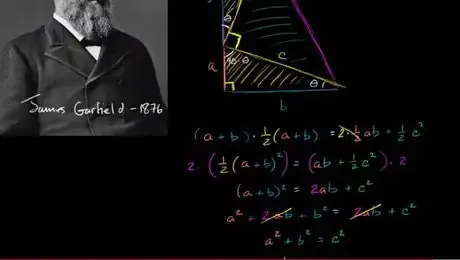
Garfield was the 20th President in 1881 and did this proof of the Pythagorean Theorem while he was still a seated member of Congress in 1876. It is interesting to note that he was fascinated by geometry, like President Lincoln, but was not a professional mathematician or geometer.
Steps
Part 1
Part 1 of 3:
The Tutorial
-
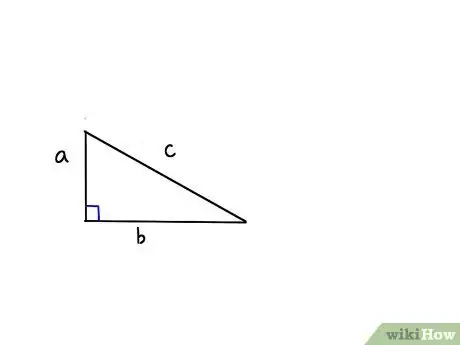
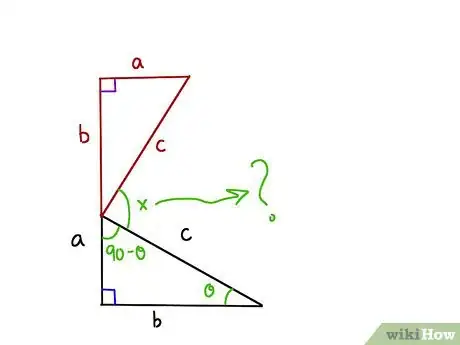
1Construct a right triangle resting on side b with right angle to the left connected to upright and perpendicular side a, with side c connecting the endpoints of a and b.,br>
-
2Construct a similar triangle with side b now extending in a straight line from the original side a, then with side a parallel along the top to the bottom original side b, and side c connecting the endpoints of the new a and b.Advertisement
-
3Understand the goal. We are interested to know the angle x formed where the two side c's meet. Thinking about it, the original triangle was made of 180 degrees with the angle on the right at the far end of b, called theta, and the other angle at the top of a, being 90 degrees minus theta, as all the angles total 180 degrees and we already have one 90 degree angle.
-
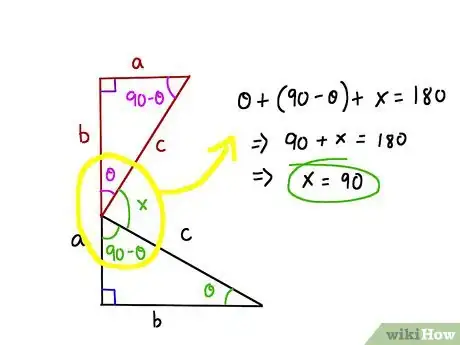
4Transfer your angle knowledge to the upper new triangle. At the bottom, we have theta, at the top left we have 90 degrees, and the top right we have 90 degrees minus theta.
- The mystery angle x is 180 degrees. So theta + 90 degrees-theta + x = 180 degrees. Adding theta and negative theta gives us zero on the left, and subtracting 90 degrees from both sides leaves x equal to 90 degrees. So we have established that the mystery angle x = 90 degrees.
-
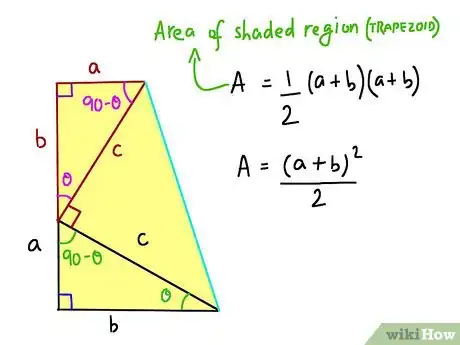
5Look at the whole figure as a trapezoid in two ways. First, the formula for a trapezoid is A= the Height x (Base1 + Base 2)/2. The height is a+b and (Base1 + Base 2)/2 = 1/2(a + b). So that all equals 1/2 (a+b)^2.
-
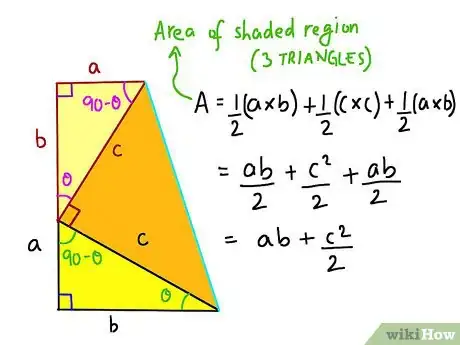
6Look at the interior of the trapezoid and add up the areas, in order to set them equal to the formula just found. We have the two smaller triangles at bottom and left, and those together equal 2*1/2(a*b), which just equals (a*b). Then we also have 1/2 c*c, or 1/2 c^2. So together we have the other formula for the area of the trapezoid equaling (a*b)+ 1/2 c^2.
-
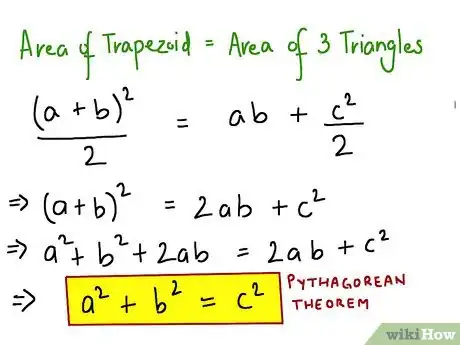
7Set the two Area formulas equal. 1/2(a+b)^2=(a*b)+1/2 c^2. Now multiply both sides by 2 to get rid of the 1/2's 2(1/2 (a+b)^2) = 2((a*b)+ 1/2 c^2.) which simplifies as (a+b)^2 = 2ab + c^2.
Advertisement
Part 2
Part 2 of 3:
Explanatory Charts, Diagrams, Photos
References
- http://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0086%3Abook%3D1%3Atype%3DProp%3Anumber%3D47 - resource source, content is shared on a Creative Commons Sharealike 3.0 license, Please refer esp. to Book I, Proposition 47
- Video: "James Garfield's proof of the Pythagorean Theorem." -khanacademy Published on Nov 27, 2012- LICENSE: Creative Commons (Attribution-Noncommercial-No Derivative Works).
About This Article
Advertisement