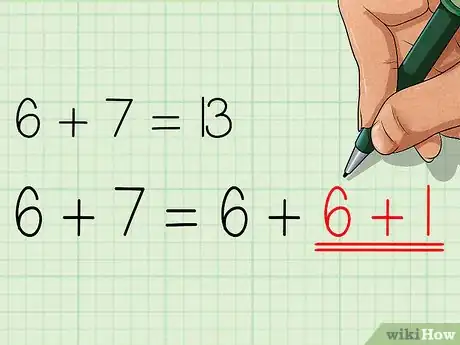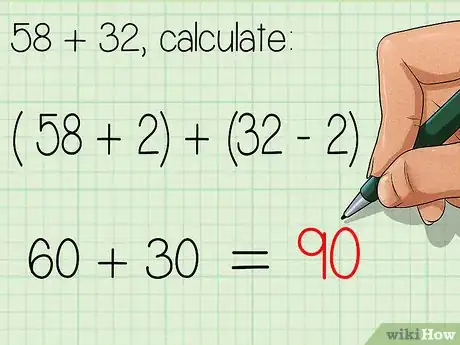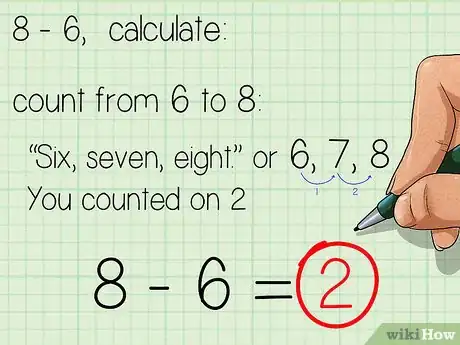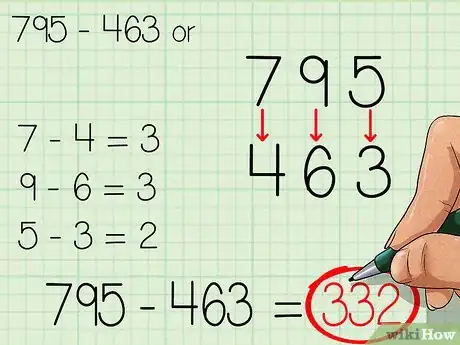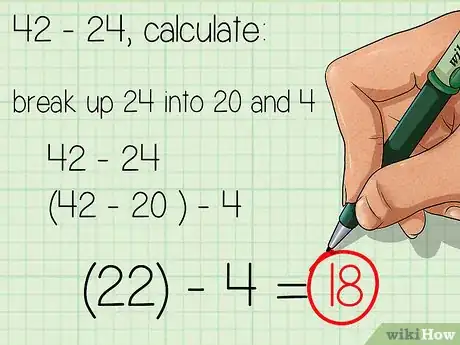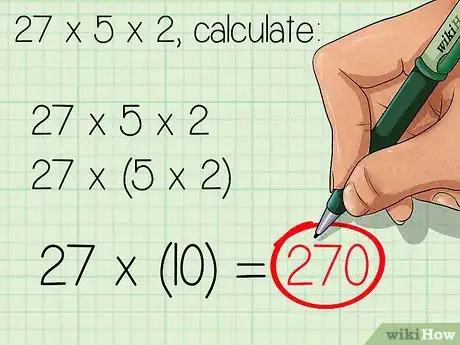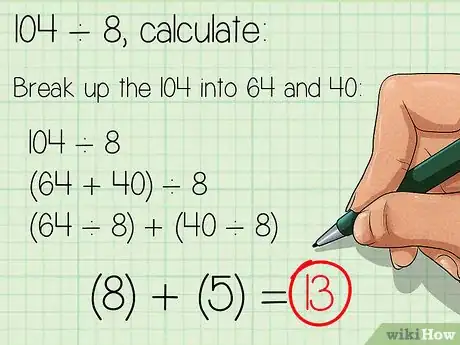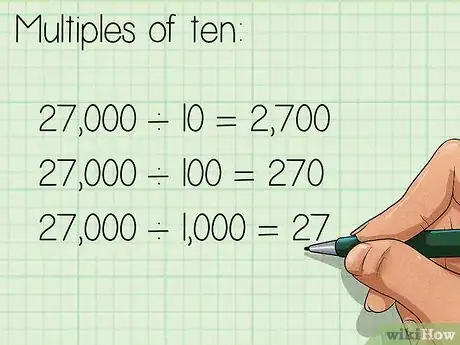How to Teach Mental Math

Co-authored by Ronitte Libedinsky, MS

Last Updated: June 29, 2020

Calculators, computers, and other electronics have changed the way educators teach mathematics. Unfortunately, our reliance on technological aids has caused many of the mental math skills taught previously to fall by the wayside. Still, it is possible to teach students math strategies that will help them quickly add, subtract, multiply, and divide mentally when these aids are not available. These are also good methods to have students use to check their work.

Part 1

Part 1 of 4:

Adding with Mental Math

$Image titled Teach Mental Math Step 1$

1
Understand the value of 0. Adding zero to a number does not change its value.
- For example, if I have 6 apples, and you have 0 apples, together we have 6 apples:
  $6+0=6$
$Image titled Teach Mental Math Step 2$

2
Understand the commutative property. The commutative property states that numbers can be added in any order.
- For example, 7 apples plus 4 apples is the same as 4 apples plus 7 apples. They both equal 11 apples:
  $7+4=4+7$
  $11=11$
Advertisement
$Image titled Teach Mental Math Step 3$

3
Add by counting on. Use the commutative property and start with the larger number, then count up the value of the smaller number.
- This strategy works best when one of the addends is less than five.
- Students can use their fingers or manipulatives to keep track of how many they count on.
- For example, to calculate $7+3$ , begin with 7 and count on three: “Seven, eight, nine, ten.”
$Image titled Teach Mental Math Step 4$

4
Make a ten when adding three or more numbers. Use the commutative property to make a ten, then add the remaining number.
- For example, to calculate $3+6+7$ , first make a ten by adding 7 and 3, then add 6:
  $3+6+7$
  $(7+3)+6$
  $(10)+6=16$
$Image titled Teach Mental Math Step 5$

5
Memorize doubles. A double is an addition sentence that adds a number to itself.
- Adding a number to itself yields a number twice as big as the original number, so if students know how to multiply by two, they can use multiplication to help them add.
- For example, students can memorize doubles up to 10:
  $1+1=2$
  $2+2=4$
  $3+3=6$
  $4+4=8$
  $5+5=10$
  $6+6=12$
  $7+7=14$
  $8+8=16$
  $9+9=18$
  $10+10=20$
$Image titled Teach Mental Math Step 6$

6
Recognize doubles plus one. A doubles plus one is an addition sentence that would be a double, except one number is one larger than the other. Once students have their doubles memorized, they can simply add 1 to the doubles sum.
- For example, if a student knows that $6+6=12$ , they can recognize that $6+7=13$ , because $6+7=6+6+1$ .
$Image titled Teach Mental Math Step 7$

7
Use skip counting. Students can use skip counting when adding by twos, fives, or tens.
- Students should recognize that any even number plus two will equal an even number, and any odd number plus two will equal an odd number.
- For example, $5+5+5$ is the same as skip counting by fives three times: “Five, ten, fifteen.”
$Image titled Teach Mental Math Step 8$

8
Think of plus 9 as plus 10 minus 1. To do this, whenever you add by 9, add by 10 instead, and then subtract 1 from the sum.
- For example, to calculate $29+9$ , calculate:
  $29+10=39$
  $39-1=38$
$Image titled Teach Mental Math Step 9$

9
Break up larger numbers to make compatible numbers. Compatible numbers are numbers that are easier to add together.
- For example, to calculate $58+32$ , you can break up 58 into $50+8$ , and you can break up 32 into $30+2$ . Then you can use the commutative property to add compatible numbers first:
  $50+8+30+2$
  $(50+30)+(8+2)$
  $80+10=90$
$Image titled Teach Mental Math Step 10$

10
Balance numbers before adding. To balance numbers, you can subtract from one number and add the same amount to the other.
- For example, to find $58+32$ , you could subtract 2 from 30, then add 2 to 58.
  $58+32$
  $(58+2)+(32-2)$
  $60+30=90$

Advertisement

Part 2

Part 2 of 4:

Subtracting with Mental Math

$Image titled Teach Mental Math Step 11$

1
Count on from the number you are subtracting (the subtrahend) to the number you are subtracting from (the minuend). The result will be the answer, or difference.
- Students can use their fingers or manipulatives to count on.
- For example, to calculate $8-6$ , start with 6 and see how many you have to count on to get to 8: “Six, seven, eight.” You counted on 2, so $8-6=2$ .
$Image titled Teach Mental Math Step 12$

2
Use the front end strategy for problems that require no borrowing. To do this, subtract the digits beginning with the largest place value, ending with the lowest place value.
- When subtracting with pencil and paper you usually start from the ones place. When using the front end strategy, you work beginning from the other direction.
- This strategy only works when you do not have to borrow from other place values. You will know that the problem requires no borrowing if, when you line up the place values of each number, all the digits you are subtracting are smaller than the digits you are subtracting from.
- For example, to calculate $795-463$ , you would first subtract the hundreds place, then the tens place, then the ones place:
  $7-4=3$
  $9-6=3$
  $5-3=2$
  So $795-463=332$ .
$Image titled Teach Mental Math Step 13$

3
Break up the subtrahend into tens and ones.^{[1]
X
Research source} Then subtract your group of tens, then subtract the group of ones.
- You can also use this strategy to break up numbers into hundreds and tens, or larger place values, for easier subtraction.
- For example, to calculate $42-24$ , break up 24 into 20 and 4:
  $42-24$
  $(42-20)-4$
  $(22)-4=18$

Advertisement

Part 3

Part 3 of 4:

Multiplying with Mental Math

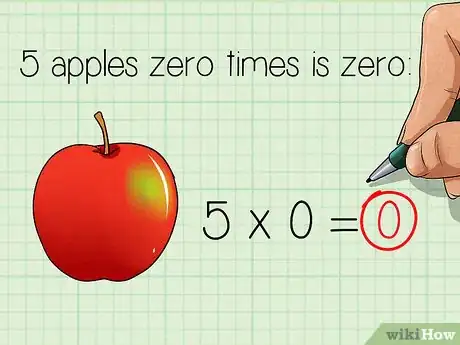
$Image titled Teach Mental Math Step 14$

1
Understand the value of 0. A number multiplied by 0 will always equal 0.
- For example, 5 apples zero times is zero: $5\times 0=0$ .
$Image titled Teach Mental Math Step 15$

2
Understand the value of 1. A number multiplied by 1 will always equal the number.
- For example, 5 apples 1 time is 5: $5\times 1=5$ .
$Image titled Teach Mental Math Step 16$

3
Use the shortcut for multiples of ten. The shortcut is that, when multiplying any number by a multiple of ten, simply add the number of zeroes in the multiple to the other number.
- For example:
  $27\times 10=270$
  $27\times 100=2,700$
  $27\times 1000=27,000$
$Image titled Teach Mental Math Step 17$

4
Use the associative property. The associative property states that you can change the order of groupings you multiply first.
- For example, to calculate $27\times 5\times 2$ , multiplying the 5 and 2 first will make a ten, which makes the problem easier:
  $27\times 5\times 2$
  $27\times (5\times 2)$
  $27\times (10)=270$
$Image titled Teach Mental Math Step 18$

5
Use the factor of 5 as half the factor of 10. To do this, whenever you are multiplying a number by 5, multiply by 10 instead, and then half the product.
- For example, to calculate $27\times 5$ , change the problem to $27\times 10$ , then divide the answer in half:
  $27\times 5={\frac {1}{2}}(27\times 10)$
  $27\times 5={\frac {1}{2}}(270)$
  $27\times 5=135$
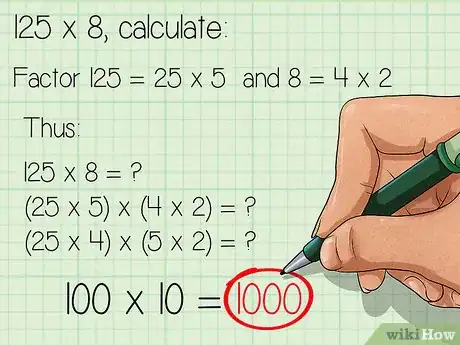
$Image titled Teach Mental Math Step 19$

6
Break up numbers into compatible factors. Compatible numbers are numbers that are easier to multiply.
- For example, to calculate $125\times 8$ , you can factor 125 as $25\times 5$ and 8 as $4\times 2$ . You can then use the commutative and associative property to multiply the factors in any order or combination. Thus:
  $125\times 8$
  $(25\times 5)\times (4\times 2)$
  $(25\times 4)\times (5\times 2)$
  $100\times 10=1000$
$Image titled Teach Mental Math Step 20$

7
Double one number and half the other. This is another way of finding compatible numbers that are easier to multiply.
- For example, to calculate $8\times 45$ , you could half the 8 and double the 45:
  $8\times 45=4\times 90$
  $8\times 45=360$

Advertisement

Part 4

Part 4 of 4:

Dividing with Mental Math

$Image titled Teach Mental Math Step 21$

1
Use the distributive property. To do this, break up the number you are dividing into smaller numbers that can easily be divided by the divisor. Then, sum the quotients.
- For example, to calculate $104\div 8$ , break up the 104 into 64 and 40:
  $104\div 8$
  $(64+40)\div 8$
  $(64\div 8)+(40\div 8)$
  $(8)+(5)=13$
$Image titled Teach Mental Math Step 22$

2
Use the shortcut for multiples of ten. The shortcut is that, when dividing any number by a multiple of ten, simply subtract the number of zeroes in the multiple from the other number.
- For example:
  $27,000\div 10=2,700$
  $27,000\div 100=270$
  $27,000\div 1,000=27$
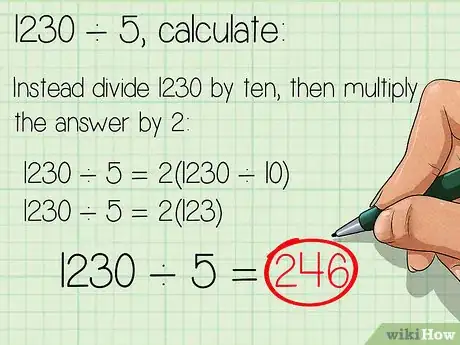
$Image titled Teach Mental Math Step 23$

3
Use the divisor 5 as half the divisor of 10. Whenever you are dividing a number by five, you can instead divide the number by ten, then multiply the quotient by 2.
- For example, to calculate $1230\div 5$ , instead divide 1230 by ten, then multiply the answer by 2:
  $1230\div 5=2(1230\div 10)$
  $1230\div 5=2(123)$
  $1230\div 5=246$

Advertisement

Expert Q&A

Question

What's a good way to improve your mental math?

Ronitte Libedinsky, MS
Academic Tutor
Ronitte Libedinsky is an Academic Tutor and the Founder of Brighter Minds SF, a San Francisco, California based company that provides one-on-one and small group tutoring. Specializing in tutoring mathematics (pre-algebra, algebra I/II, geometry, pre-calculus, calculus) and science (chemistry, biology), Ronitte has over 10 years of experience tutoring to middle school, high school, and college students. She also tutors in SSAT, Terra Nova, HSPT, SAT, and ACT test prep. Ronitte holds a BS in Chemistry from the University of California, Berkeley, and an MS in Chemistry from Tel Aviv University.

Ronitte Libedinsky, MS

Academic Tutor

Expert Answer

Try using a website like Quizlet to help you practice. You can also use a deck of cards at home to practice your mental math. Pick an operation to work on, like multiplication. Then, lay down 2 cards next to each other face-up, and solve the problem in your head.

Advertisement

You Might Also Like

Advertisement

References

↑ http://www.homeschoolmath.net/teaching/a/subtract_mentally_2_digit.php
↑ Ronitte Libedinsky, MS. Academic Tutor. Expert Interview. 26 May 2020.
↑ Ronitte Libedinsky, MS. Academic Tutor. Expert Interview. 26 May 2020.

About This Article

Co-authored by:

Ronitte Libedinsky, MS

Academic Tutor

This article was co-authored by Ronitte Libedinsky, MS. Ronitte Libedinsky is an Academic Tutor and the Founder of Brighter Minds SF, a San Francisco, California based company that provides one-on-one and small group tutoring. Specializing in tutoring mathematics (pre-algebra, algebra I/II, geometry, pre-calculus, calculus) and science (chemistry, biology), Ronitte has over 10 years of experience tutoring to middle school, high school, and college students. She also tutors in SSAT, Terra Nova, HSPT, SAT, and ACT test prep. Ronitte holds a BS in Chemistry from the University of California, Berkeley, and an MS in Chemistry from Tel Aviv University. This article has been viewed 30,274 times.

14 votes - 100%

Co-authors: 13

Updated: June 29, 2020

Views: 30,274

Categories: Teaching Mathematics

Advertisement