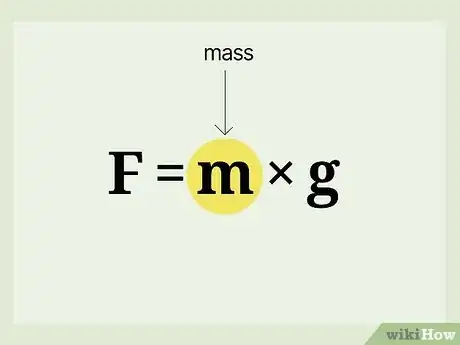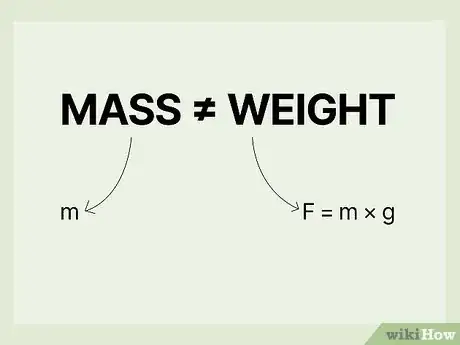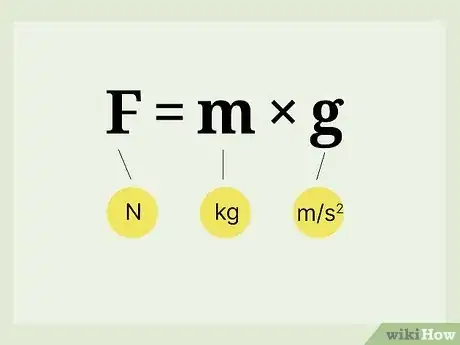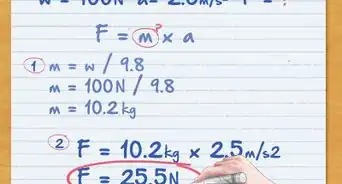This article was co-authored by wikiHow staff writer, Johnathan Fuentes. Johnathan Fuentes is a writer based in the New York City region. His interests as a writer include space exploration, science education, immigration, Latinx cultures, LGBTQ+ issues, and long-form journalism. He is also an avid hiker and has backpacked in Alaska and Newfoundland, Canada. A son of Cuban immigrants, he is bilingual in English and Spanish. Prior to joining wikiHow, he worked in academic publishing and was a freelance writer for science websites. He graduated from Columbia University in 2021, where he studied nonfiction writing and wrote for the student newspaper. He is currently counting down the seconds until the release of Kerbal Space Program 2 in 2023—a game that will almost certainly take up what little free time he has.
This article has been viewed 1,240,422 times.
Learn more...
If you’re taking a physics class, you’ll probably be asked to calculate weight from mass. But how do you do this, exactly? We’ve got you covered. While it sounds tricky, calculating weight from mass is very straightforward if you know which formula to use. This article will teach you that formula, plus how to use it when you encounter physics problems in class. As a bonus, we’ve included some practice problems to help these concepts sink in. Keep reading to learn how to calculate weight from mass on your next physics quiz, test, or homework assignment.
Things You Should Know
- The weight of an object equals the force of gravity exerted on that object. The mass of an object is always the same, but its weight changes depending on gravity.
- Use the formula to calculate weight from mass. In this formula, = weight (in N), = mass (in kg), and = acceleration due to gravity (in m/s2).
- Since weight is a force, scientists also write the equation as , where = force (in N), = mass (in kg), and = acceleration due to gravity (in m/s2).
- The value of gravity, , is 9.8 m/s2 on Earth. Its value is different elsewhere in the universe. For example, on the moon, = 1.622 m/s2.
Steps
Weight from Mass Calculation Help
Calculating Weight
-
1Use the formula to convert weight into mass. Weight is defined as the force of gravity on an object. Scientists put that sentence into an equation by writing , or .[1]
- Since weight is a force, scientists also write the equation as .[2]
- = symbol for weight, measured in Newtons, N.
- = symbol for mass, measured in kilograms, or kg.
-
= symbol for gravitational acceleration, expressed as m/s2, or meters per second squared.
- If you're using meters, the gravitational acceleration at the Earth's surface is 9.8 m/s2. Always use m/s2 for acceleration, unless you’re instructed to do otherwise.
- If you're asked to use feet, instead of meters, the gravitational acceleration on Earth is 32.2 ft/s2. This is the same unit, it's just converted from meters to feet. Luckily, you’re very unlikely to encounter a problem with acceleration written in ft/s2.
-
2Determine the mass of the object. Because we're trying to get weight from mass, we can assume we already have the mass. Mass is the fundamental amount of matter an object has, and is expressed in kilograms.Advertisement
-
3Determine the gravitational acceleration. In other words, figure out what is. On the surface of the Earth, is 9.8 m/s2. Elsewhere in the universe, the acceleration of gravity is different.[3]
- The gravitational acceleration on the moon is different from the gravitational acceleration on the Earth. Acceleration due to gravity on the moon is about 1.622 m/s2, or about 1/6 of the acceleration that it is here on Earth. That's why you weigh 1/6 of your Earth-weight on the moon.[4]
- The gravitational acceleration on the sun is different from the gravitational acceleration on the Earth and moon. Acceleration due to gravity on the sun is about 274.0 m/s2, or about 28 times the acceleration that it is here on Earth. That's why you would weigh 28 times your Earth-weight on the sun (if you could survive!).[5]
-
4Plug the numbers into the equation . Now that you've got and , plug those values into the equation . You’ll get a number described in Newtons, or N.
Sample Problems
-
1Example #1: An object has a mass of 100 kg. What is its weight on the surface of the Earth?
- We know equals 100 kg, and equals 9.8 m/s2 (since this is the gravitational acceleration on Earth’s surface).
- Plug these values into the equation: = 100 kg * 9.8 m/s2.
- = 980 N. In other words, on the surface of the Earth, an object with a mass of 100 kg will weigh about 980 Newtons on Earth.
-
2Example #2: An object has a mass of 40 kg. What is its weight on the surface of the moon?
- We know equals 40 kg, and equals 1.6 m/s2 (since the gravitational acceleration on the Moon’s surface is 1.6 m/s2).
- Plug these values into the equation: = 40 kg x 1.6 m/s2.
- = 64 N. In other words, on the surface of the moon, an object with a mass of 40 kg will weigh approximately 64 Newtons on the moon.
-
3Example #3: An object weighs 549 Newtons on the surface of the Earth. What is its mass?
- For this problem, we have to work backward. We have and , but we need to calculate .
- Set up the equation: 549 = * 9.8 m/s2.
- Instead of multiplying * , we divide by . In other words, .
- Plugging in our values, we get = 549/9.8 m/s2.
- = 56 kg. In other words, an object weighing 549 Newtons on the surface of the Earth will have a mass of about 56 kilograms.
Catching Mistakes
-
1Avoid confusing mass and weight. Remember that mass is the amount of "stuff" in an object, which stays the same no matter where you move it. Weight measures the force of gravity on that "stuff," which changes if you move through space. Try these mnemonic devices to help you memorize your units:
- Mass is in units of grams or kilograms. Both mass and gram contain an . Weight is in units of newtons. Both weight and newton contain a w.
- You only have weight while you're "wait"ing on Earth, but even "mass"tronauts have mass.
-
2Always use scientific units: kg, N, and m/s2. Most physics problems use newtons (N) for weight, meters per second squared (m/s2) for gravitational force, and kilograms (kg) for mass. If you use a different unit for one of these values, your calculations will be incorrect. Convert all values to scientific units before plugging them into the standard equation. These conversions may help you out if you're used to the imperial / U.S. system:
- 1 pound-force = ~4.448 newtons
- 1 foot = ~0.3048 meters
Community Q&A
-
QuestionHow do you find mass with just force
 wikiHow Staff EditorThis answer was written by one of our trained team of researchers who validated it for accuracy and comprehensiveness.
wikiHow Staff EditorThis answer was written by one of our trained team of researchers who validated it for accuracy and comprehensiveness.
Staff Answer wikiHow Staff EditorStaff AnswerThis is not possible. The equation you'd use is F=ma, where F = the force (in N), m = mass (kg), and a = acceleration (in m/s^2). To calculate mass of an object from a known force, you'll need to know the acceleration of that object.
wikiHow Staff EditorStaff AnswerThis is not possible. The equation you'd use is F=ma, where F = the force (in N), m = mass (kg), and a = acceleration (in m/s^2). To calculate mass of an object from a known force, you'll need to know the acceleration of that object. -
QuestionHow do I get the gravitational force and multiply it by the mass?
 Community AnswerGravitational force is proportional the two bodies' masses and the gravitational constant and is inversely proportional to the square of the distance between them. F= (m1 (Earth)*m2 (observed body)*G(constant))/r/r. G= 6.67408 × 10-11 m3 kg-1 s-2. However, for the sake of weight we usually take the gravitational accelration (g) which is equal to 9.81 m/s/s, usually rounded to 10 m/s/s. G (gravitational force/weight) = g (gravitational accelration) * m (mass of the observed body). If you use both methods, the two Gs should be equal barring some rounding errors.
Community AnswerGravitational force is proportional the two bodies' masses and the gravitational constant and is inversely proportional to the square of the distance between them. F= (m1 (Earth)*m2 (observed body)*G(constant))/r/r. G= 6.67408 × 10-11 m3 kg-1 s-2. However, for the sake of weight we usually take the gravitational accelration (g) which is equal to 9.81 m/s/s, usually rounded to 10 m/s/s. G (gravitational force/weight) = g (gravitational accelration) * m (mass of the observed body). If you use both methods, the two Gs should be equal barring some rounding errors. -
QuestionDoes a scale measure weight or mass?
 Community AnswerIt measures weight, because it measures the force gravity exerts on an object. But since we know what the gravitational constant is on Earth, we can convert that weight into mass (and many scales simply show the mass because they're calibrated to do so).
Community AnswerIt measures weight, because it measures the force gravity exerts on an object. But since we know what the gravitational constant is on Earth, we can convert that weight into mass (and many scales simply show the mass because they're calibrated to do so).
Addendum: Weights Expressed in kgf
- A Newton is a SI-unit. Quite often the weight is expressed in kilogramforce or kgf. This is not a SI-unit, therefore less impeccable. But it is very convenient for comparing weights anywhere with weights on Earth.
- 1 kgf = 9.8166 N.
- Divide the calculated number of Newtons by 9.80665, or use the last column when available.
- The weight of the 101 kg astronaut is 101.3 kgf on the North Pole, and 16.5 kgf on the moon.
- What is an SI-unit? It stands for Systeme International d'Unites, a complete metric system of units of measurement for scientists.
References
- ↑ https://www.grc.nasa.gov/www/k-12/VirtualAero/BottleRocket/airplane/wteq.html
- ↑ https://web.mst.edu/~reflori/be150/Dyn%20Lecture%20Videos/F=ma%20Particle%20Straight%20Line%201/F=ma%20Particle%20Straight%20Line%201.pdf
- ↑ https://www.physicsclassroom.com/class/1DKin/Lesson-5/Acceleration-of-Gravity
- ↑ https://hypertextbook.com/facts/2004/MichaelRobbins.shtml
- ↑ https://www.smartconversion.com/otherInfo/gravity_of_planets_and_the_sun.aspx
About This Article
To find weight when you already know the mass, use the formula weight = mass times gravitational acceleration. Remember that on the surface of the earth, gravitational acceleration is always 9.8 m/s^2, so simply plug in the mass and multiply it by 9.8 to get the weight in newtons. For sample problems and tips for checking your answer and catching mistakes, read on!